
ยินดีต้อนรับ ทุกท่านเข้าสู่ เว็บแทงหวยชั้นนำอันดับ 1 ที่ดีที่สุดในประเทศไทย 2021
999LUCKY112 เว็บแทงหวยอออนไลน์อินเตอร์ โดยเรามีบริการ แทงหวยออนไลน์ มี ระบบ ฝาก-ถอน รวดเร็ว เว็บแทงหวยเปิดให้บริการนานกว่า 10 ปี จึงมั่นใจได้ถึง ความปลอดภัยและ มีมาตรฐานการบริการ 24 ชั่วโมง !
โดยเฉพาะ หวยหุ้นออนไลน์ เราเป็นหนึ่งในผู้ให้บริการรับ แทงหวยหุ้น ที่ดีที่สุด มีหวยจากทุกประเทศ ออกผลตรงเวลา ไม่ต้องรอนานราคา อัตราจ่ายสูงที่สุด พร้อมโปรโมชั่นส่วนลดมากกว่าที่ไหนๆ เว็บแทงหวยจ่ายจริงต้องเราเท่านั้น 999LUCKY112
สมัครแทงหวยออนไลน์ กับเราคุณจะได้รับการให้บริการที่เป็นเลิศ เรามีเจ้าหน้าที่คอยให้คำปรึกษา หากท่านเกิดปัญหาใดๆ สามารถสอบถามได้ทันที และ ที่สำคัญสามารถเข้าเล่น หวยออนไลน์ ได้อย่างสบายใจไร้กังวล เรามีมาตรฐาน ความปลอดภัยสูงสุด เราไม่มีนโยบายเปิดเผยข้อมูลของลูกค้า ดังนั้นข้อมูลของท่านจะถูกเก็บเป็นความลับ อย่างแน่นอน อยากเล่นหวย หุ้นออนไลน์ หวยรัฐบาล ต้องเลือกเล่น หวยออนไลน์
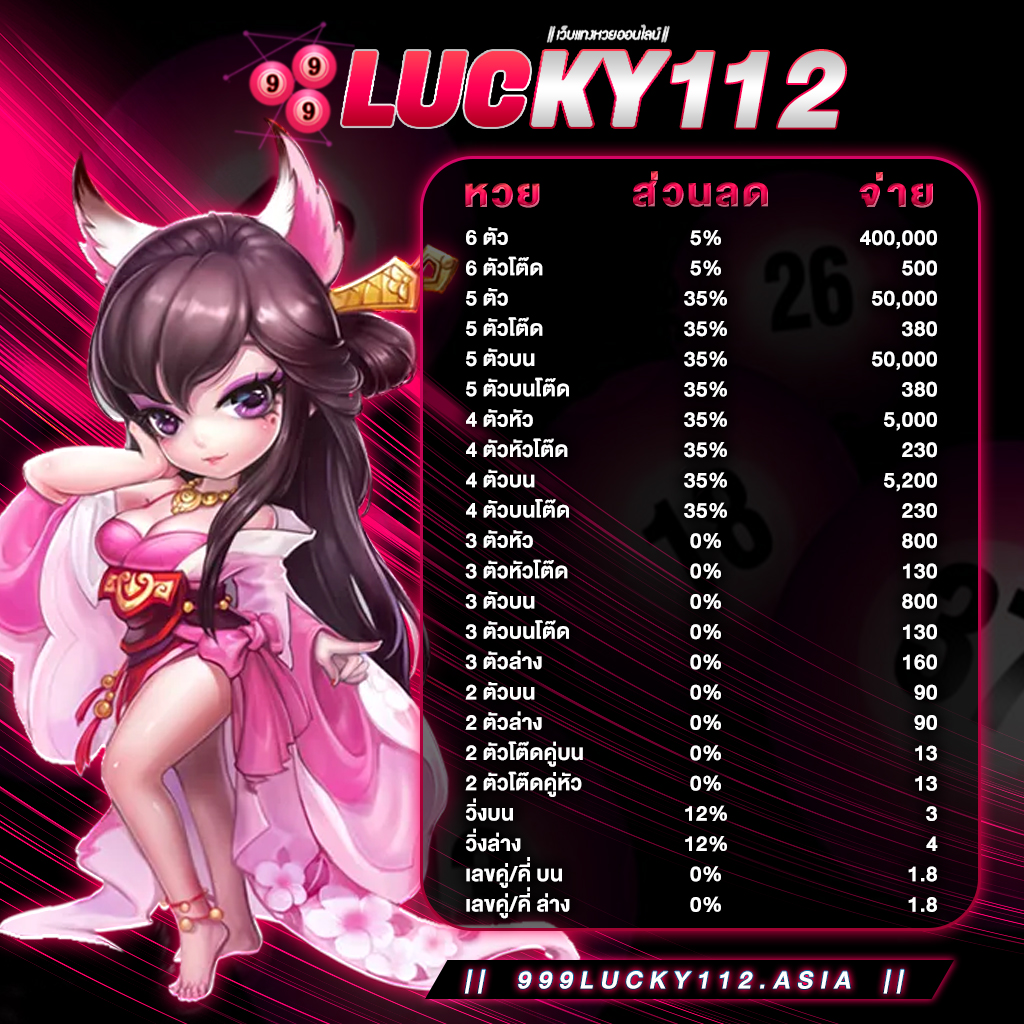
เปิดให้บริการแล้ว ส่วนลดสูงที่สุด 33% จ่ายสูงที่สุด ถึง 550
โปรโมชั่นหวยลดสูงสุด 33% จ่ายมากที่สุด 550 แทงหวยปลอดภัยมั่นใจ
3 ตัว ลดสูงสุด 33% จ่าย 550 บาท
2 ตัวบนและล่าง ลดสูงสุด 28% จ่าย 70 บาท
3 ตัวล่าง จ่าย 125 บาท
วิ่งอัตราลด 12%
3 ตัวโต๊ด จ่าย 105 บาท
2 ตัวโต๊ด จ่าย 12 บาท
แทงขั้นต่ำเพียง 10 บาทเท่านั้น
ซื้อสลากกินแบ่ง แทงหวย ขายล๊อตเตอรี่ สลากกินแบ่งออนไลน์ สลากกินแบ่งหุ้นออนไลน์ สลากกินแบ่งไทย ล๊อตเตอรี่หุ้นไทย ดาวโจนส์ ดาวโจนส์ หุ้นไทย สลากกินแบ่ง ออนไลน์ หวยรัฐบาล สลากกินแบ่งหุ้น เลขเด็ด ลุ้นมั่งคั่ง สูตรหุ้น huay999 เลขเด็ด เลขราว เลขชุด สลากกินแบ่งหุ้นต่างชาติ สลากกินแบ่งยี่กี่ และก็ ฯลฯ พร้อมระบบฝาก-ถอน เร็วทันใจด้านใน 5 นาที พร้อมคณะทำงานระดับประสิทธิภาพ ได้เงินจริง ที่เว็บไซต์ดีมีคุณภาพ มาแรงที่สุดในปี จะต้องที่ 999lucky เพียงแค่นั้น !
ตรวจสลากกินแบ่งออนไลน์ หวยรัฐบาล ล๊อตเตอรี่ลาว สลากกินแบ่งฮานอย ล๊อตเตอรี่หุ้น ตรวจผลสลากกินแบ่งฟรี ทุกสลากกินแบ่ง เพียงท่านสมัครเป็นสมาชิกเว็บไซต์สลากกินแบ่งออนไลน์ กับพวกเรา ก็สามารถ แทงหวยได้ราคายอดเยี่ยมในไทย
ตรวจสลากกินแบ่งฟรี ทุกงวดแถมเข้าพวกไลน์แจก เลขเด็ดฟรี ลงทะเบียน สมัครสมาชิก ตรงนี้ไม่มีผิดหวัง ทุกๆยอดแทงตั้งแต่ 50 ขึ้นไป ยังได้ Reward 1 คะแนนเพื่อเก็บไว้ใช้แทงแจ็คพ็อต 6 ตัว ลุ้นได้จากรางวัลสลากกินแบ่งหวยรัฐบาล วันแล้ววันเล่าที่ 1 แล้วก็ 16 ของทุกงวด อีกด้วย คุ้มขนาดนี้ อย่ารอคอยช้า รีบสมัครกันเลย
แทงหวยออนไลน์2021 กับ เว็บหวยออนไลน์ชั้นนำยอดนิยม จ่ายไม่ยั้ง ต้องการมั่งคั่ง ไม่รู้ตัว ต้องมาที่ 999LUCKU112 เท่านั้น ครับ !
อย่ากลัวการแทงหวย แนวทางการลงทะเบียนสิ่งจำเป็นที่จะต้องมีก่อนที่จะเล่น ล๊อตเตอรี่ออนไลน์ อันดับแรกพวกเรามาเช็คมองว่าพวกเราควรมีอะไรก่อน จำเป็นต้องจัดเตรียมอะไรที่อยู่ในการเล่น หรือ ลงทะเบียนเว็บไซต์ บัญชีธนาคารไทย เป็นชื่อของท่านเอง เพื่อความสบายสำหรับเพื่อการ ฝาก-ถอน เบอร์โทรศัพท์มือถือจริงของท่าน ที่ใช้งานประจำ ในบางครั้ง แม้กำเนิดปัญหา บุคลากรจะติดต่อเพื่อช่วยเหลือท่านตลอด 24 ชั่วโมง !
เว็บหวยออนไลน์มาแรง2021 ที่ครบวงจรที่ดีที่สุด ได้รับการยอมรับมากที่ สุดสำหรับนักเสี่ยงโชคในไทยมี หวยออนไลน์ ให้ท่านเลือกเล่นมากมาย คัดสรรค์หวยที่ดีที่สุดทั่วโลกมาให้ท่านได้เล่นกันอย่างจุใจ เเละเพลิดเพลิน ครับ สนใจสมัครแทงหวย !
และไม่ว่าจะเป็น หวยรัฐบาล หวยจับยี่กี หวยหุ้นไทย หวยหุ้นต่างประเทศ หวยสิงคโปร์ หวยลัคกี้เฮง หวยฮานอยเช้า หวยฮานอยบ่าย หวยฮานอยดิจิตอล หวยมาเลย์พิเศษ หวยมาเลกลันตัน หวยมาเล ดิจิตอล หวยมาเลเช้า หวยมาเลบ่าย หวยมาเล 5G หวยลาว VIP หวยลาวพิเศษ หวยลาว5D
หวยนิเคอิ Vip หวยนิเคอิ พิเศษ หวยนิเคอิ ดิจิตอล หวยฮังเส็ง Vip หวยฮังเส็งพิเศษ หวยฮังเส็งดิจิตอล เป็นต้น และยังมีเกมส์ คาสิโนออนไลน์ อีกมากมาย อาทิเช่น เสือมังกร ปั่นแปะ เป่ายิ้งฉุบ สล็อต รูเร็ต บาคาร่า และเกมส์กีฬาอื่นๆ อีกมากมาย ท่านสมาชิกสามารถ ติดต่อ-สอบถาม !
จุดเด่นของ เว็บหวยออนไลน์ 999LUCKY คือเปิดบริการรับแทงหวย ซื้อหวยออนไลน์ชั้นนำ กับเราเพราะมีความมั่นคง ด้านการเงินปลอดภัย 100% แถมยังมี อัตราการจ่าย สูงถึงบาทละ 800 ส่วนลดเยอะจ่ายเงินแล้ว เล่นได้เงินจริง จ่ายเต็ม ไม่มีโกง แน่นอนครับ !
ทำไมต้องเลือก แทงเว็บหวยออนไลน์ 999LUCKY112
- ง่ายต่อการซื้อ เเละ แถมยังสะดวกสบาย
- เลขดัง ซื้อได้ไม่อั้น เเละ ยังสามารถซื้อได้ถึง 15.30
- หากถูกราลวัลระบบจะตัดเงินให้ทันที
- มีอัตราการจ่ายเยอะกว่า เว็บ อื่นๆ
- สำคัญ จ่ายจริง จ่ายไว ไม่โกงมั่นคงปลอดภัย 100%
- ข้อมูลส่วนตัวและข้อมูลด้านบัญชีของทุกท่านจะถูกเก็บไว้เป็นความลับสูงสุด ทางบริษัทจะไม่นำข้อมูลแก่บุคคลอื่นทุกกรณี
- ความปลอดภัยจากทางบริษัทเราใช้ระบบความปลอดภัยที่มีเสถียรภาพสูง มั่นใจได้
- เราจะรักษาความปลอดภัยของทุกท่านไว้อย่างดีแน่นอน โดยเฉพาะข้อมูลส่วนตัวของทุกท่าน
- บริษัทเราให้บริการมาหลายปี จึงมีความน่าเชื่อถือสูงทำให้ลูกค้าที่มาเล่นกับเราทุกคน อุ่นใจในการเล่น
- หากท่านมีข้อสงสัยใดๆ สามารถติดต่อ ฝ่ายบริการลูกค้า ได้ตลอด 24 ชั่วโมง
สมัครสมาชิกฟรีวันนี้…ลุ้นรับสิทธิพิเศษ มากมายจากทางเว็บพร้อม โปรโมชั่น สำหรับลูกค้าใหม่ เท่านั้น !
สมัครสมาชิก
เข้าสู่ระบบ
วิธีฝาก-ถอน
ติดต่อ-สอบถาม
ทางเข้าแทงหวย 999LUCKY
สำหรับท่านลูกค้าท่านใดที่กำลังมองหา เว็บแทงหวยที่ดีที่สุด ในตอนนี้ผมจึงขอนำเสนอ เว็บแทงหวยชั้นนำ 999LUCKY112 การันตรี ความปลอดภัย มั่นคง 100% ครับท่าน !
ติดต่อทีมงาน 999LUCKY ได้ตลอด 24 ชั่วโมง / บริการงานโดย เฮียบิ๊ก @ ปอยเปต
999LUCKY112 เว็บแทงหวยชั้นนำอันดับ 1 ในประเทศไทย 2021
แถมยังมีระบบ ฝาก-ถอน อัตโนมัต มั่นคง ปลอดภัย เเละจ่ายจริงไม่โกง 100 %
หวยยี่กี| หวยออนไลน์| หวยรัฐบาล| หวยฮานอย| หวยมาเล





